EXPONENTIAL SPEED ADVANTAGE
SCALABILITY ACROSS SYSTEM SIZES
ENHANCED DATA REPRESENTATION
ENERGY EFFICIENT COMPUTATION
What is Quantum Mechanics ?
Quantum mechanics is a branch of physics that explores how matter and energy behave at the smallest scales, such as electrons, atoms, and even particles of light (photons). It’s different from everyday physics because it deals with phenomena that are hard to visualize: particles that seem to be in multiple places at once, communicate instantly, and pass through solid objects!
How Does Quantum Mechanics Work?
Instead of thinking of particles as tiny balls, quantum mechanics treats them as “waves” of possibility. This means they don’t have one fixed position but a range of positions until we measure them. It’s like knowing that a cat is either inside or outside a box, but in the quantum world, it’s both—until you check!
Why is Quantum Mechanics Important?
Quantum mechanics allows us to explore complex systems that classical physics struggles to explain.
Here’s how it’s used:
1. Quantum Chemistry: Modeling chemical reactions to design better drugs or materials by simulating the behavior of complex molecules like proteins, enzymes, and catalysts at the quantum level.
2. Quantum Computing: Solving problems faster by using particles to simulate large molecules, such as carbon nanotubes or metal-organic frameworks, to accelerate material discovery and molecular design.
3. Quantum Cryptography: Creating unbreakable codes to protect data by leveraging the entanglement of photons to secure molecular-level research and sensitive biochemical information.
What Makes Quantum Mechanics Special?
In classical physics, if you drop a ball, you know exactly where it will land. In quantum mechanics, particles don’t follow such straightforward rules. They “choose” paths based on probability, so instead of one predictable outcome,
you get a range of possible outcomes.
Why Groupe Curie Uses Quantum Mechanics ?
At Groupe Curie, we use the unique properties of quantum mechanics to solve scientific problems that would take classical methods far longer or make them impossible. From modeling new chemical compounds to optimizing industrial processes,
our work focuses on using these advanced principles to push industries forward and create a sustainable future.
Quantum Mechanics: Scientific Foundations and Innovations in CURIE-MATRIX™
The CURIE-MATRIX™ leverages fundamental quantum principles, such as superposition, entanglement, and tunneling, to unlock computational capabilities far beyond classical systems. In classical computing, each bit
represents a single state (0 or 1), while quantum systems can exist in multiple states simultaneously, thanks to superposition. This parallelism allows CURIE-MATRIX™ to explore a vast number of solutions
at once. Furthermore, entanglement creates strong correlations between qubits, enabling rapid data exchange. These properties, combined with advanced error correction, make the CURIE-MATRIX™ exceptionally
powerful for solving high-dimensional problems efficiently.
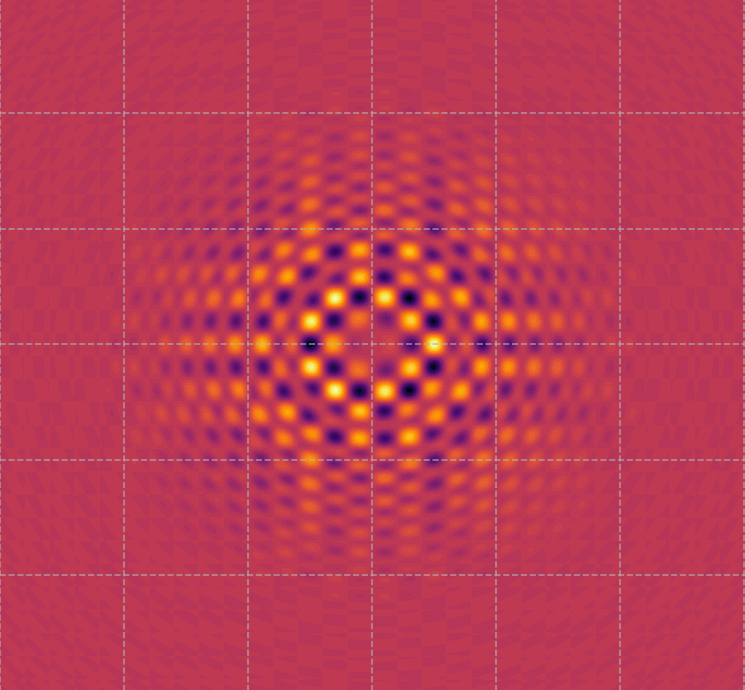
Visualization of the wave function or surface states of a topological insulator.
Topological insulators are unique materials where the surface states conduct electricity, but the bulk remains insulating. This image represents the
distribution of electron density or the wave interference patterns of these surface states, highlighting the
unique symmetry and behavior of electrons in topological insulators.
These patterns are what make topological insulators special, as they are highly resistant to impurities and defects, allowing electrons to move along the surface without scattering. This property has significant implications for
quantum computing and spintronics (what makes elaboration of data much faster than traditional systems that needs to pass over a logical gate operation).

Electron Probability Density of the Hydrogen Atom’s Ground State
This image is a representation of the electron probability density for a hydrogen atom in its ground state (the 1s orbital). The bright red spot in the center indicates the highest probability of finding the electron, which is located
near the nucleus.
The plot’s circular symmetry reflects the spherically symmetric nature of the hydrogen atom’s ground state. As the distance from the nucleus increases, the probability density decreases smoothly, which is consistent with the radial
distribution function of a hydrogen atom.
This visualization is a fundamental example in quantum mechanics, illustrating how the electron’s position is described by a wave function rather than a fixed orbit.
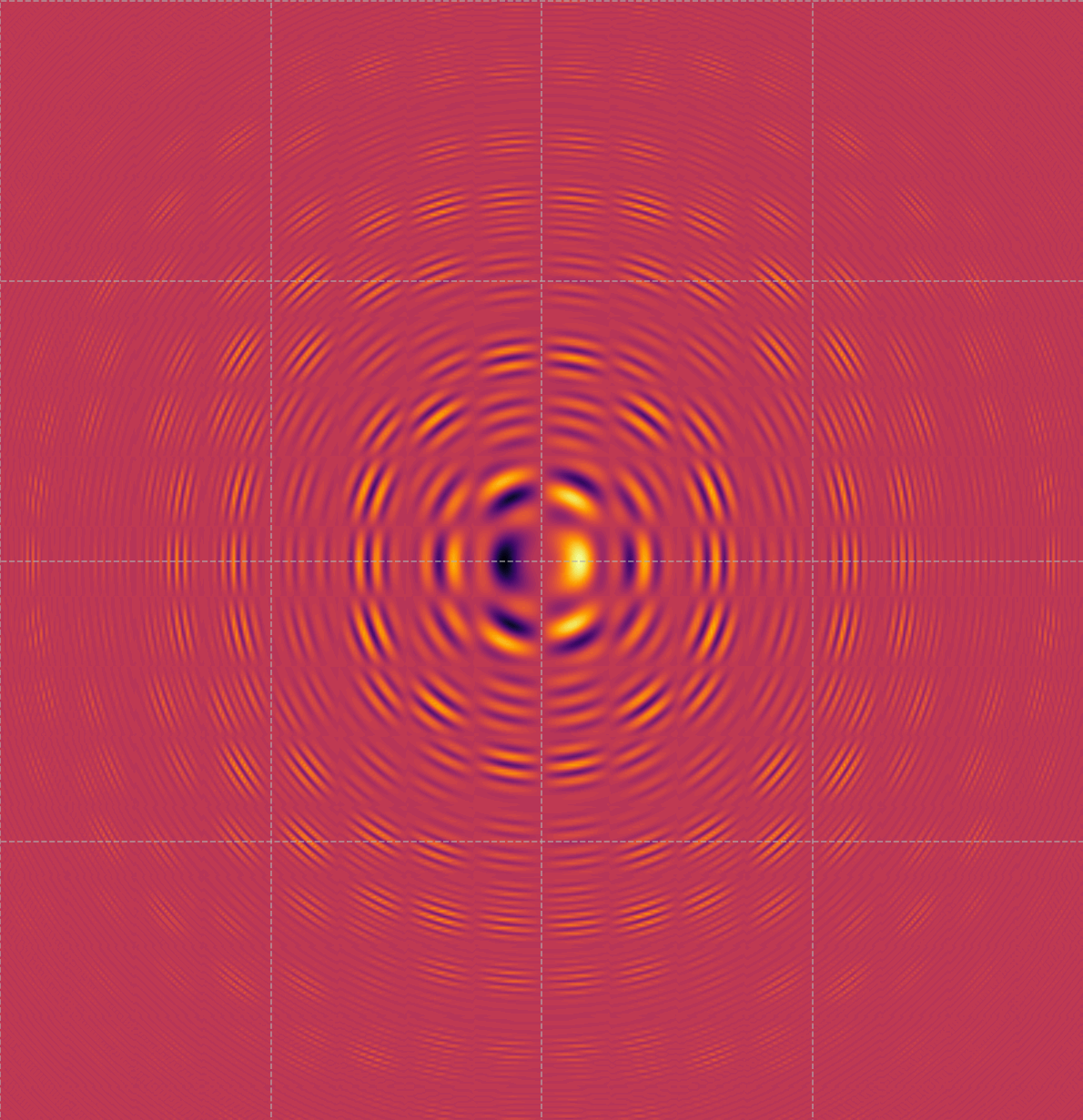
Visualizing Quantum Probability Densities in a Symmetric Potential
This image displays a quantum interference pattern, most likely a visualization of the probability density of a wave function in a symmetric potential well. The pattern of concentric rings and alternating bright and dark areas indicates regions of high and low probability for locating a particle, such as an electron, within a confined system. The central bright spot represents the area with the highest probability, while the rings indicate the wave-like behavior of the particle, showing how the probability density oscillates as you move away from the center. This type of pattern can arise in scenarios such as electron orbitals in atoms, scattering patterns, or confinement in nanostructures. It provides insight into the wave nature of particles, emphasizing how quantum mechanics differs from classical expectations by illustrating superposition, standing wave patterns, and quantization. Understanding these visualizations is fundamental for analyzing the behavior of particles in quantum systems and optimizing their properties for applications in quantum computing, material science, and nano-engineering.
Quantum Mechanics in Everyday Life
Quantum Mechanics in Everyday Life: Why Does It Matter?
Quantum mechanics isn’t just a theory for scientists in labs – it’s the foundation of many technologies we use every day! Here are some examples of how quantum principles impact your life:
1. Smartphones and Computers
The miniaturization of computer chips and the speed of processors are possible because of quantum physics. Transistors, the tiny switches that power electronics, rely on quantum effects to function properly.
2. LED Lights and Lasers
Quantum mechanics explains how light is emitted by LEDs and how lasers work, which are used in everything from DVD players to medical equipment.
3. MRI Machines in Hospitals
Magnetic Resonance Imaging (MRI) scans use the quantum property of spin in atoms to create detailed images of our bodies, allowing doctors to diagnose diseases more accurately.
4. GPS Systems
Quantum mechanics helps keep the atomic clocks in satellites precise, ensuring that GPS systems can pinpoint your location accurately.
5. Future Technologies
Technologies like quantum computing and quantum communication will revolutionize fields such as cryptography, drug discovery, and artificial intelligence, making once-impossible solutions a reality.
Why This Matters for You ?
Understanding and harnessing quantum mechanics opens up endless possibilities – from more efficient energy solutions to faster data processing and safer communication. At Groupe Curie, we’re committed to turning these possibilities into practical, impactful technologies that benefit industries and society as a whole.

- SWITZERLAND
- Groupe Curie AG
- Pflanzschulstrasse 3
- 8400 Winterthur
- SWITZERLAND
- Email: info@groupe-curie.com
- Phone: +41 58 100 09 77
- Web: www.groupe-curie.com
- SWITZERLAND
- Groupe Curie Cleantech
- Kemptpark 12
- 8310 Kemptthal
- SWITZERLAND
- Email: cleantech@groupe-curie.com
- Phone: +41 58 100 09 74
- Web: www.groupe-curie.com
- ITALY – MILAN (OFFICE)
- Groupe Curie
- Corso Venezia 12
- 20121 Milan
- ITALY
- Email: info@groupe-curie.com
- Phone: +39 02 829 415 90
- Web: www.groupe-curie.eu
- ITALY – NAPLES
- Groupe Curie
- Via Raffaele Morghen 36
- 80129 Naples
- ITALY
- Email: info@groupe-curie.com
- Phone: +39 081 189 205 06
- Web: www.groupe-curie.eu
- POLAND
- Groupe Curie Sp.z.o.o
- Research & Development
- 71-497 Szczecin
- Poland
- Email: info@groupe-curie.com
- Web: www.groupe-curie.com
- ITALY – NUSCO
- Groupe Curie
- Industrial Hub
- Industrial Area Nusco
- 83051 – Nusco
- Email: info@groupe-curie.com
- Web: www.groupe-curie.eu
- ITALY – TRIESTE
- Groupe Curie Ltd.
- Planning Office
- Via Tor San Piero 30
- 34135 – Trieste
- Email: info@groupe-curie.com
- Web: www.groupe-curie.eu
Groupe Curie™ an d Curie-Matrix™ with the trademark No.018678626 and No.784349 is protected by Swiss, European and international law.
Any reproduction and usage of any branded material or data from Groupe Curie will be prosecuted by law.















